Here is a short video of the spiral sculpture created by John Edmark.
This is an example of a spidron. A spidron is a continuous geometric figure composed entirely of the scaled copies of the same polygon it is made of.
First spidron created by Dániel Erdély in 1979, consisting of equilateral triangles and obtuse isosceles triangles which together form right triangles. It was homework for the design lesson Ernő Rubik (the creator of the Rubik's Cubes)
Follow the instructions below to make your own Spidron model:
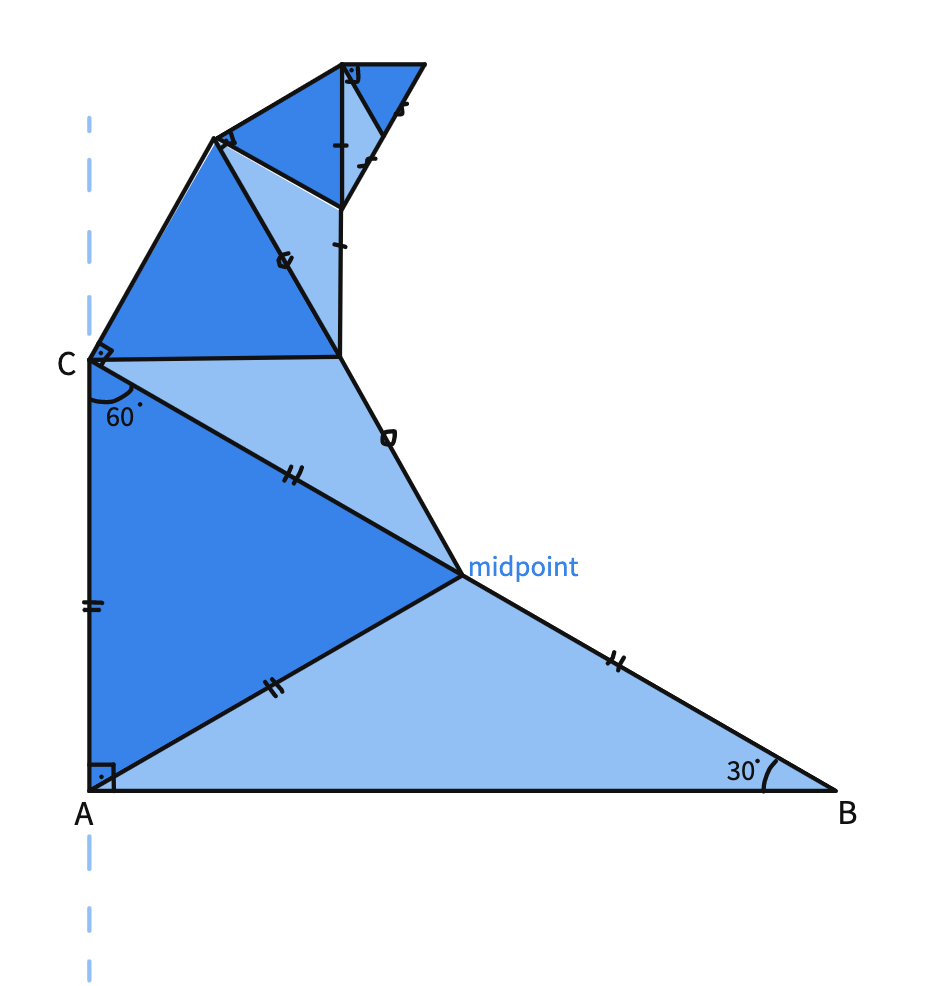
- Open a blank canvas and draw a 30-60-90 right triangle by using the ruler and the protractor tools under the utensils.
- Draw a line segment AB.
- Use the Construction tools to draw a perpendicular line to AB from A.
- Use the protractor and connect point B with point C on the perpendicular line to create a 30-degree angle between AB and BC.
- Divide the right triangle into an equilateral and isosceles triangles.
- Use the construction tools to construct the midpoint of BC.
- Connect A and C.
What are the interior angles of the isosceles triangle?
- Construct exactly the same shapes (at least in four different sizes) with the size of the new equilateral triangle's side length as the half of the hypotenuse of the previous right triangle.
Once you have created a Spidron with at least four right triangles, you can connect them as pairs or as groups of 6 to create a hexagon.
A Spidron example made by grouping as 6 to create a hexagon

Which other shapes can you see?
What is the scale factor between the two consecutive shapes?
Can Spidrons be used to tile an entire plane?
Extension
If you enjoyed this, consider exploring Rep-tiles, Fractals, and Penrose Tiles.
Resources
From the article "Some Surprising New Properties of the Spidrons" by Dániel Erdély
"In addition to the fact that the movements of the edges and faces of the spidron formations are interesting, this stable deformation may furnish ideas for many practical uses. It has been raised repeatedly that several layers of spidron reliefs could be used as shock dampers or crumple zones in vehicles. Its space-filling properties make it suitable for the construction of building blocks or toys. The surface could be used to create an adjustable acoustic wall or a system of solar cells that follow the sun in a simple manner. Various folding buildings and static structures could also be developed on the basis of my geometric investigation which may have utility in space travel."