Overview and Objective
In this activity, students will explore different nets of a cube while also investigating edge, face, and vertex properties of cubes.
Warm-Up
Start by inserting a cube into a blank canvas from the 3D solids collection of Polypad. Click here to learn more about using the nets. Talk about the number of square faces, edges, and vertices. You can use the points and lines to highlight the edges and vertices of the cube. Adjusting the transparency of the cube helps students to see these details better.
Use the unfold option to show the net of the cube. Talk about this T-shape arrangement of six squares as just one of the possible nets that can be folded up to a cube.
Perhaps the most well-known cubes used every day are dice. Roll a standard die using the Polypad dice tool and ask about the top and the bottom faces of the die. Students explore that the opposite sides of a standard die must add up to 7. Use this canvas to ask the students to create the net of a standard die using colored squares.
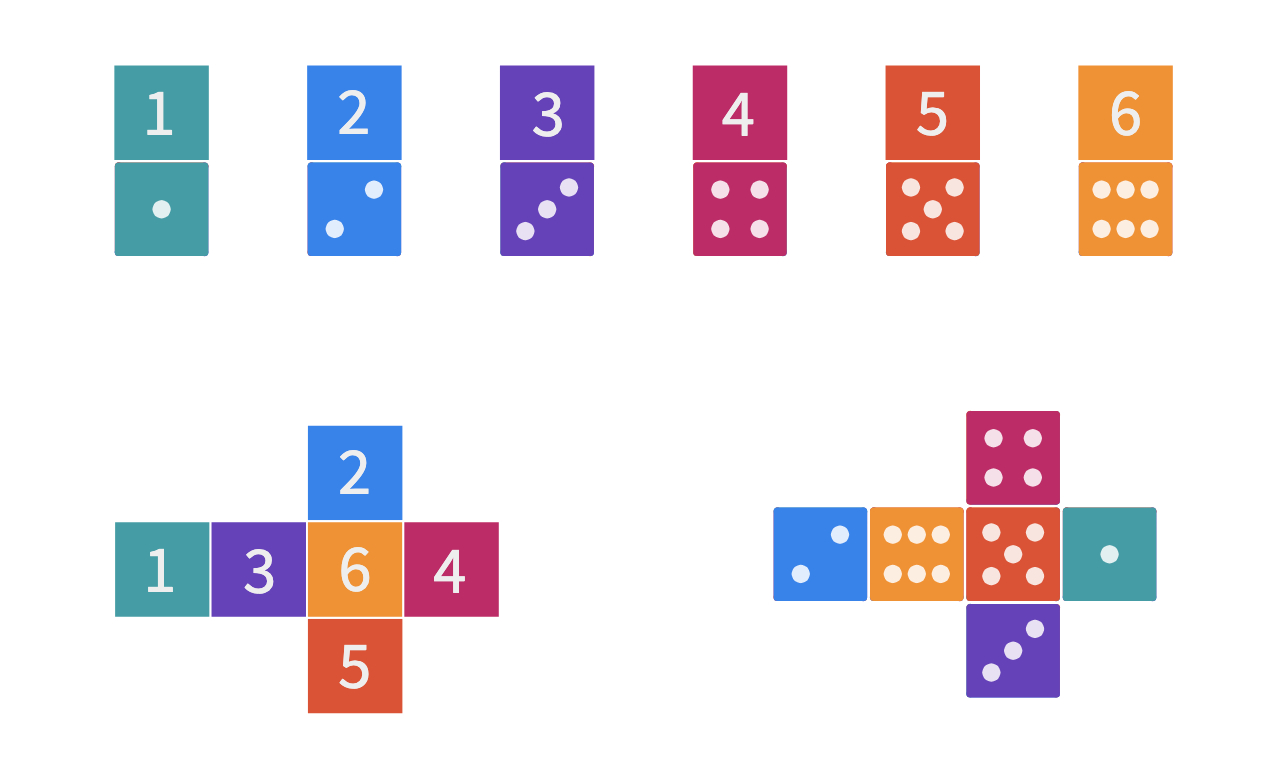
Main Activity
A cube has 6 square faces. Ask students if they think that means that any set of 6 squares can fold up a cube? One of the most well-known nets of a cube is the T shape used in the warm-up to build a fair die. What other set of 6 squares can fold up a cube?
Start by showing a rectangular arrangement of squares and discuss as a class if it is possible to fold it. If the students already knew about tiling and tessellation, you may want to discuss the sum of angles at each vertex is 360 degrees, therefore this arrangement covers a plane without gaps and overlaps, and therefore folding is not possible.
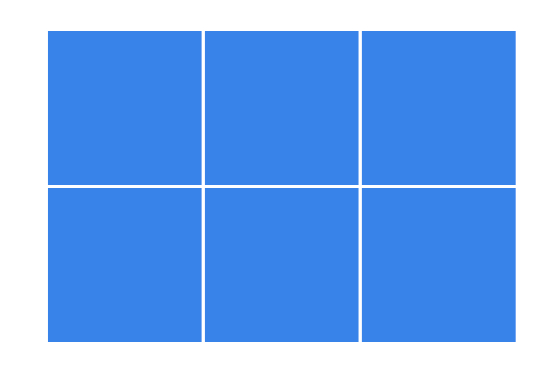
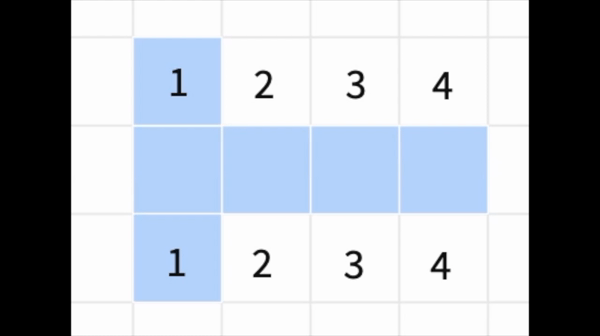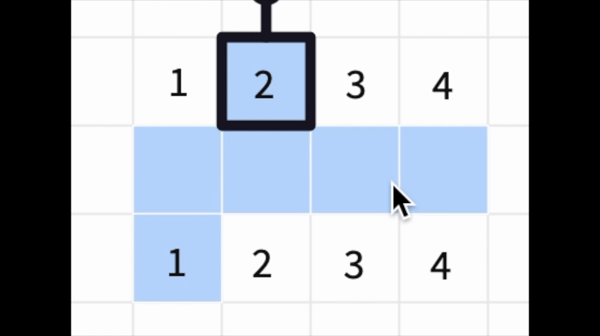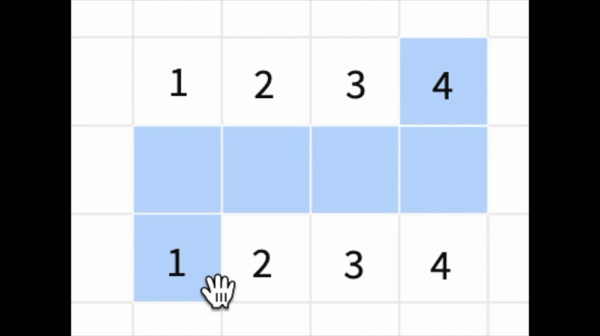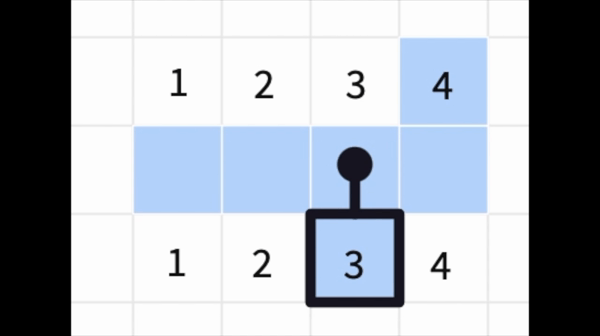
Show 1 x 6 rectangular arrangement of the squares. You may use different colors for the square faces to show how some of the faces coincide when folding them up.
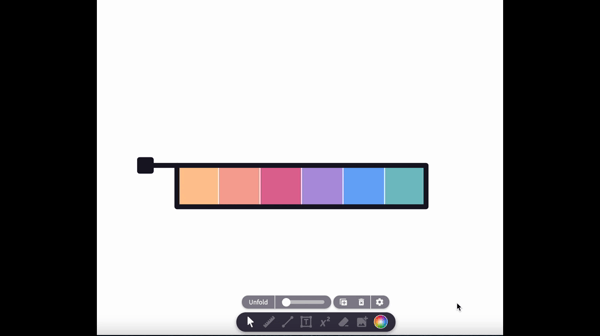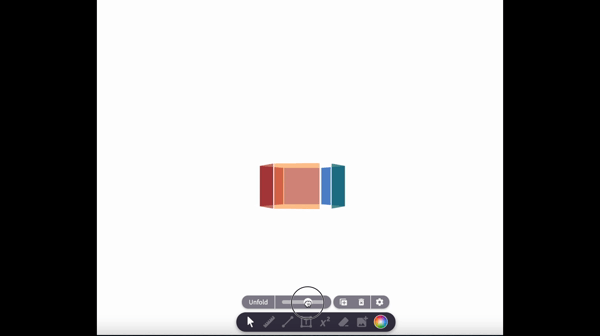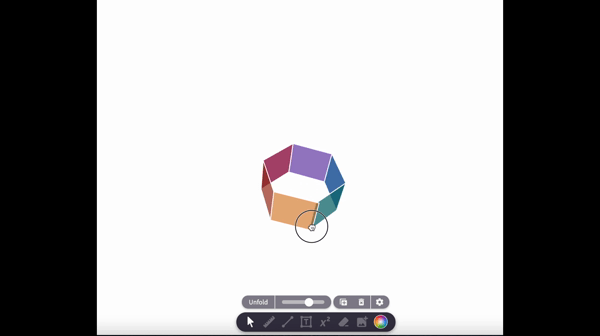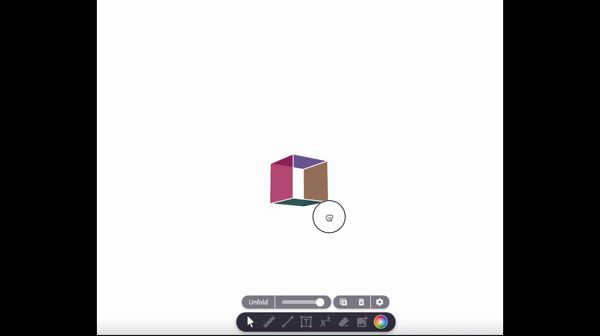
At this point, you may want to ask students the number of faces that meet at each vertex of the cube. They can also compare the T-shape net with two counterexamples to conclude 3 squares need to meet at a vertex when you fold the net of a cube.
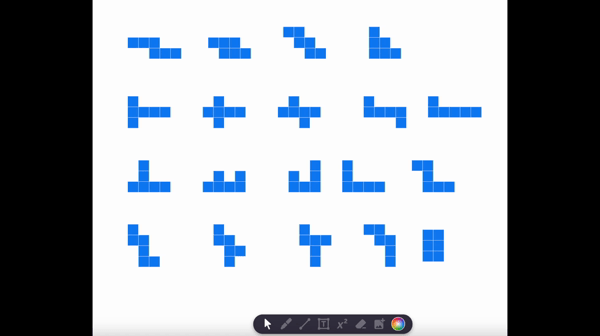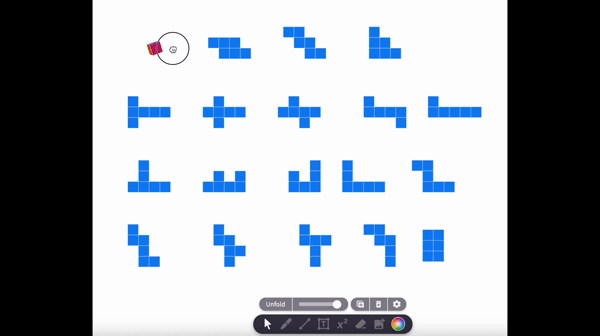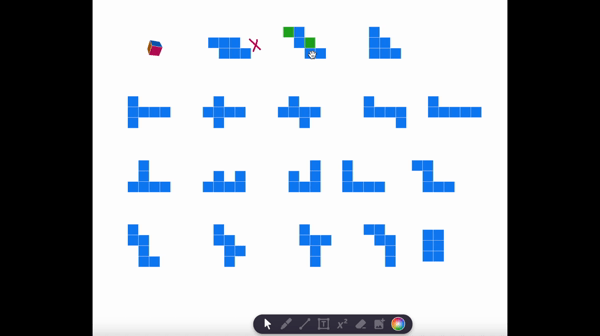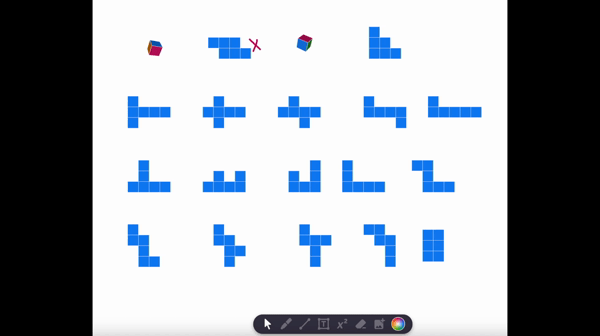
Place the students in pairs or groups of three and share this canvas and let them work on which one of these sets fold up to a cube. Alternatively, consider simply asking each group to find all possible arrangements of 6 cubes that can make a net. If you select this approach, you don't need to share a starting canvas with them.
Ask students to color the opposite faces of the cube the same color. After some work time, share some student work with the class. Use the folding the net option to check the coloring.
Closure
At the end of the lesson, assign each group a different net to design a standard die. Share some student work with the class. Some possible answers here are;
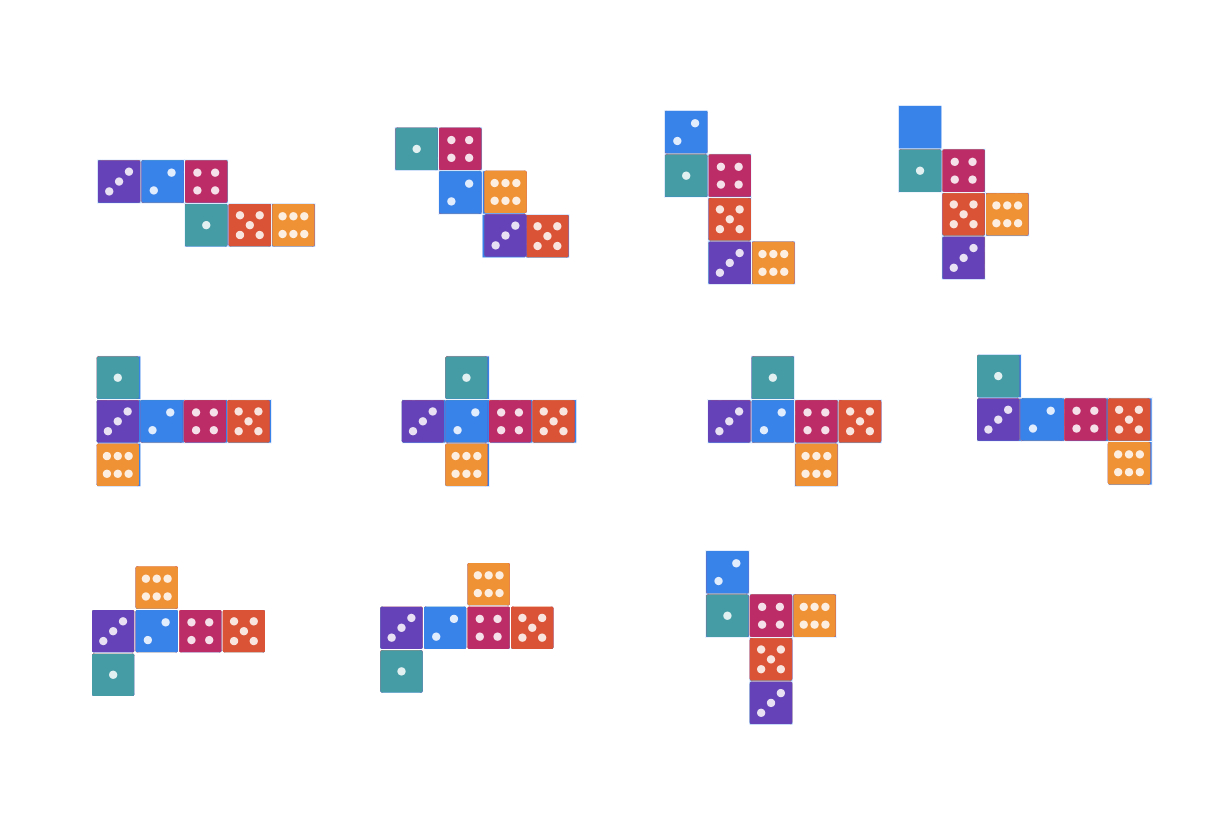
You may also end the lesson with a mini-puzzle using this canvas.
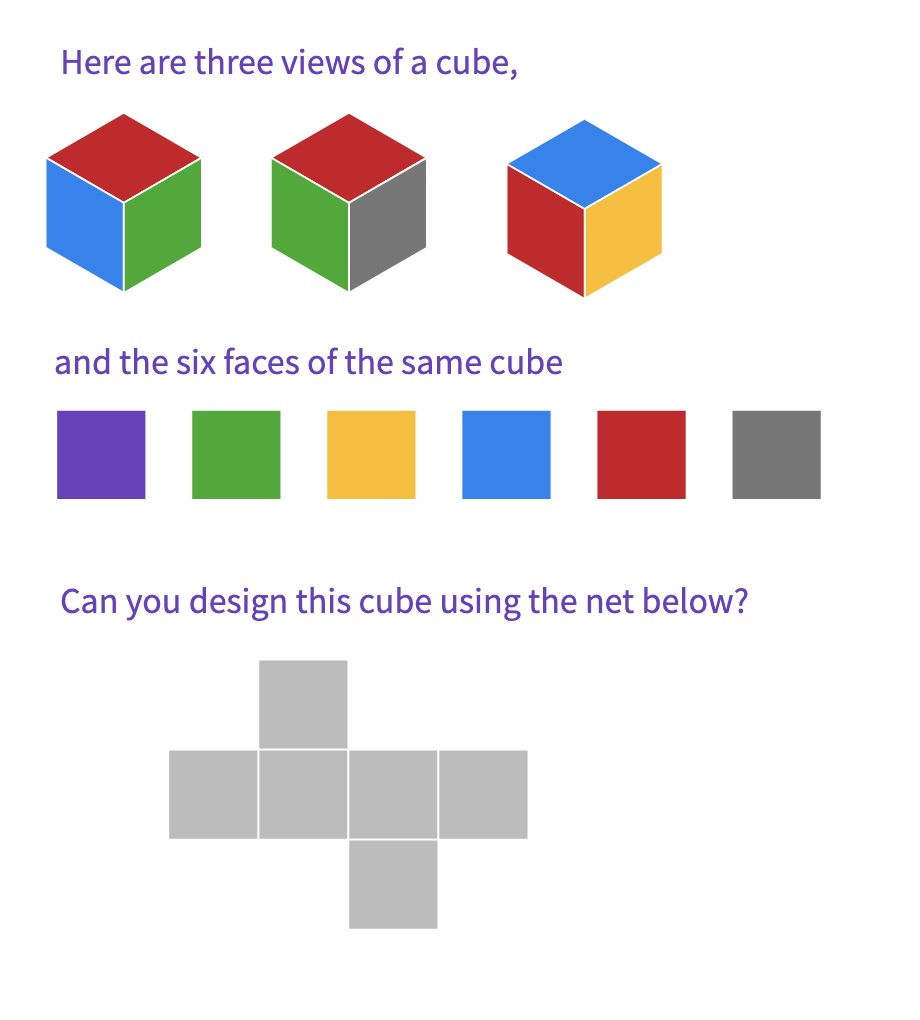
Here is a possible answer.

Support and Extension
For students needing additional support, the fold-up option can be used before coloring the opposite faces for some particular nets. For instance, when one t-shape example is shown, students can change the orientation of the caps to create 5 more nets.
As an extension, consider asking the possible nets of a tetrahedron, and a square pyramid.
Polypad for This Lesson
To assign these to your classes in Mathigon, save a copy to your Mathigon account. Click here to learn about saving a Polypad into your account and sharing it with your students.