Arrange the 4 pieces below so they fit perfectly inside the triangle.
Here's a hint if you need it:
Now rearrange the pieces into the same triangle using the new outline shown in the 2nd triangle:
What is happening here? The triangle didn't get bigger and the pieces stayed the same size. Yet, we have an empty square in the triangle!

The exploration is called Curry's Paradox. A paradox is a seemingly contradictory situation. Here, we have the same 4 shapes that seem to have different area based on how we arrange them. That doesn't seem to fit with our understanding of area. Can you explain what is happening here? Mathematician Haskell Curry created this paradoxical situation.
Solution
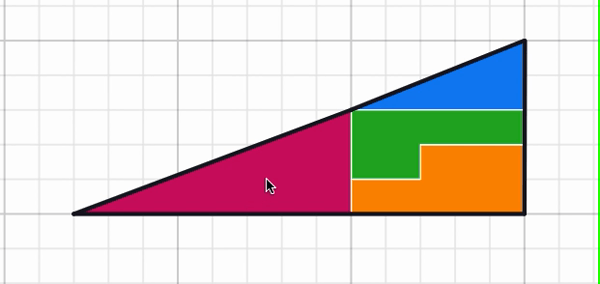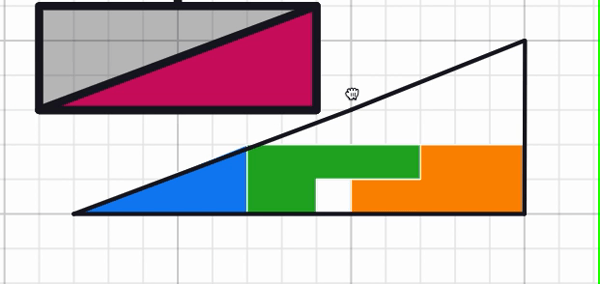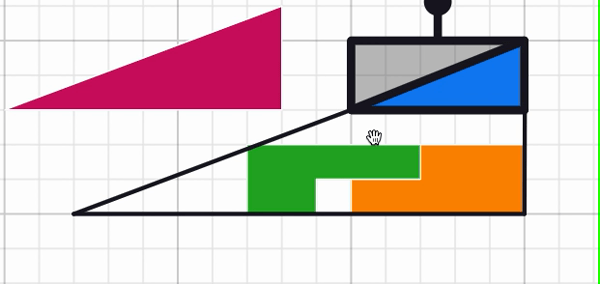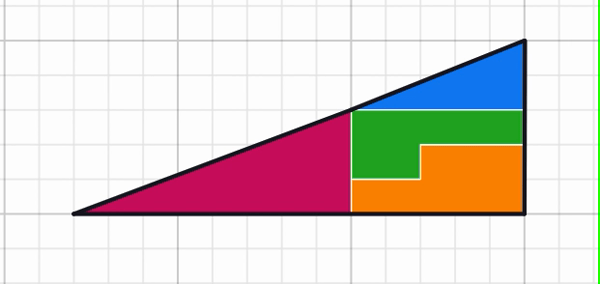
Let's start making sense of this by finding the area of the triangle and the shapes.
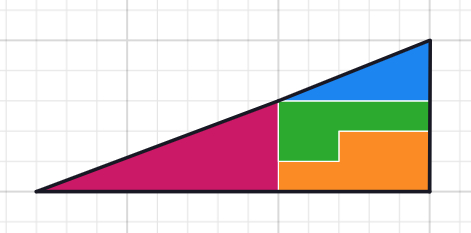
We've found the issue! The triangle has an area of 32.5 while the four pieces have an area of 32. That must mean when arranged in this manner, the 4 pieces don't quite fill up the triangle:
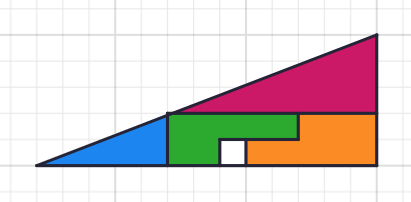
And when arranged like this, the 4 pieces plus the extra square have an area of 33. So they must be extending beyond the triangle slightly:
The blue triangle and the red triangle do NOT make a straight line. To see this, drag the blue triangle on top of the red triangle and you'll see the longest sides (the hypotenuse) don't line up exactly. Zoom in as needed.
Here is a close-up:
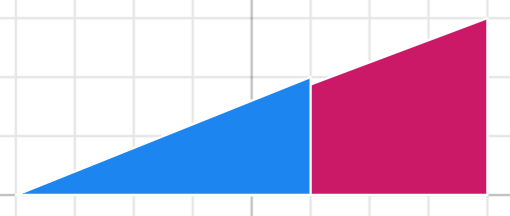
The blue triangle goes up 2 units for every 5 units over, while the red triangles goes up 3 units for every 8 units over. does not equal . These triangles have different steepness. Further, , which confirms that the hypotenuse of the blue triangle is steeper.
The large black triangle goes up 5 units for every 13 units over:
So, the steepness of the black triangle is in between the steepness of the blue and red triangle.
Below are two pictures of VERY exaggerated versions of this situation:
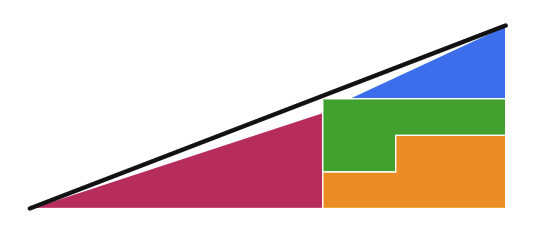
In this picture, the 4 shapes don't fill up the triangle.
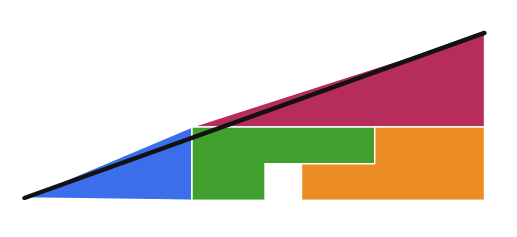
In this picture, the 4 shapes overflow outside of the triangle.
Extension
Can you make up a paradox of your own?