Egyptian Fractions

Overview and Objective

In this lesson, students explore how Ancient Egyptians wrote fractions 4000 years ago. They compare the unit fractions and express any given fraction as the sum of unit fractions. Working on the task will help students improve their skills in adding and subtracting fractions.

This activity can be used with younger students by working on the numerical examples also with the older students by finding general rules to create the unit fraction sums.

Warm-Up

The Ancient Egyptians used symbols called hieroglyphs to represent letters and numbers.

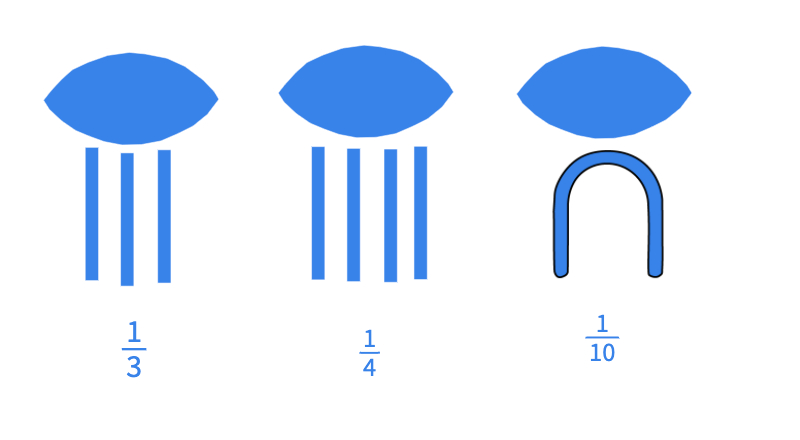
They used a single bar | for 1, two bars || for 2, $∩$ for 10, and other symbols for the powers of 10 until a million.

Show students this photo from Karnak temple, Luxor, Egypt, and ask them to read the numbers here.

Egyptians also wrote fractions using hieroglyphs. They put an oval (mouth symbol) meaning “part" above the number symbol to indicate the fraction.

Share this canvas with the students and ask them to write the numbers using hieroglyphs.

With this notation, Ancient Egyptians could write all the unit fractions.

But the most amazing part is they could also write ALL the fractions using the unit fractions. You may ask the students if they can guess how.

Main Activity

Start by reminding students that a unit fraction is a fraction whose numerator is 1. It represents one of the equal parts of a whole. The Ancient Egyptians only used symbols to write unit fractions, so any other fraction had to be represented as a $sum$ of unit fractions.

Some define Egyptian fractions as the sum of distinct unit fractions. For example, $\frac{5}{6}$ can be written as the sum of $\frac{1}{2}$ and $\frac{1}{3}$ , as well as the sum of five $\frac{1}{6}$s. But using five symbols is not very efficient since the symbols had to be carved in stone! Plus, using all these symbols requires a lot of space as well. So the Egyptian way of writing $\frac{5}{6}$ is

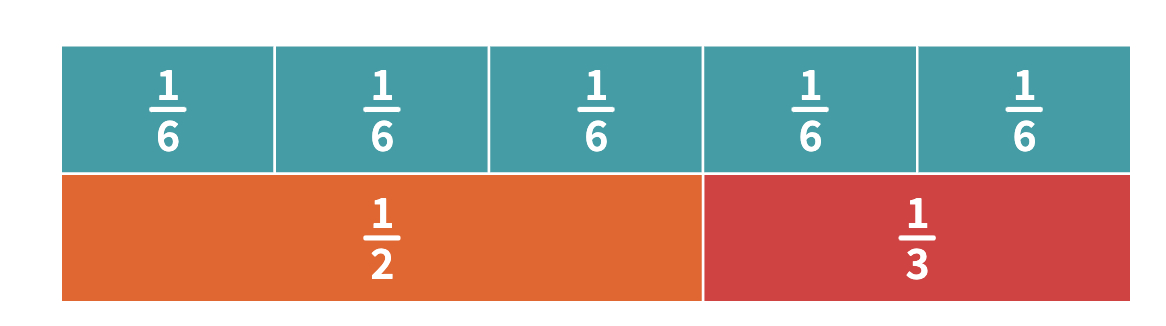
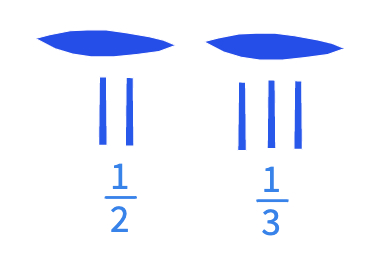
You may also discuss that $\frac{5}{6}$ can also be written as the sum of unit fractions in a different way, but that will require four symbols.

Ask students to predict if all the fractions can be written as the sum of unit fractions. Have students explore this question on this canvas.

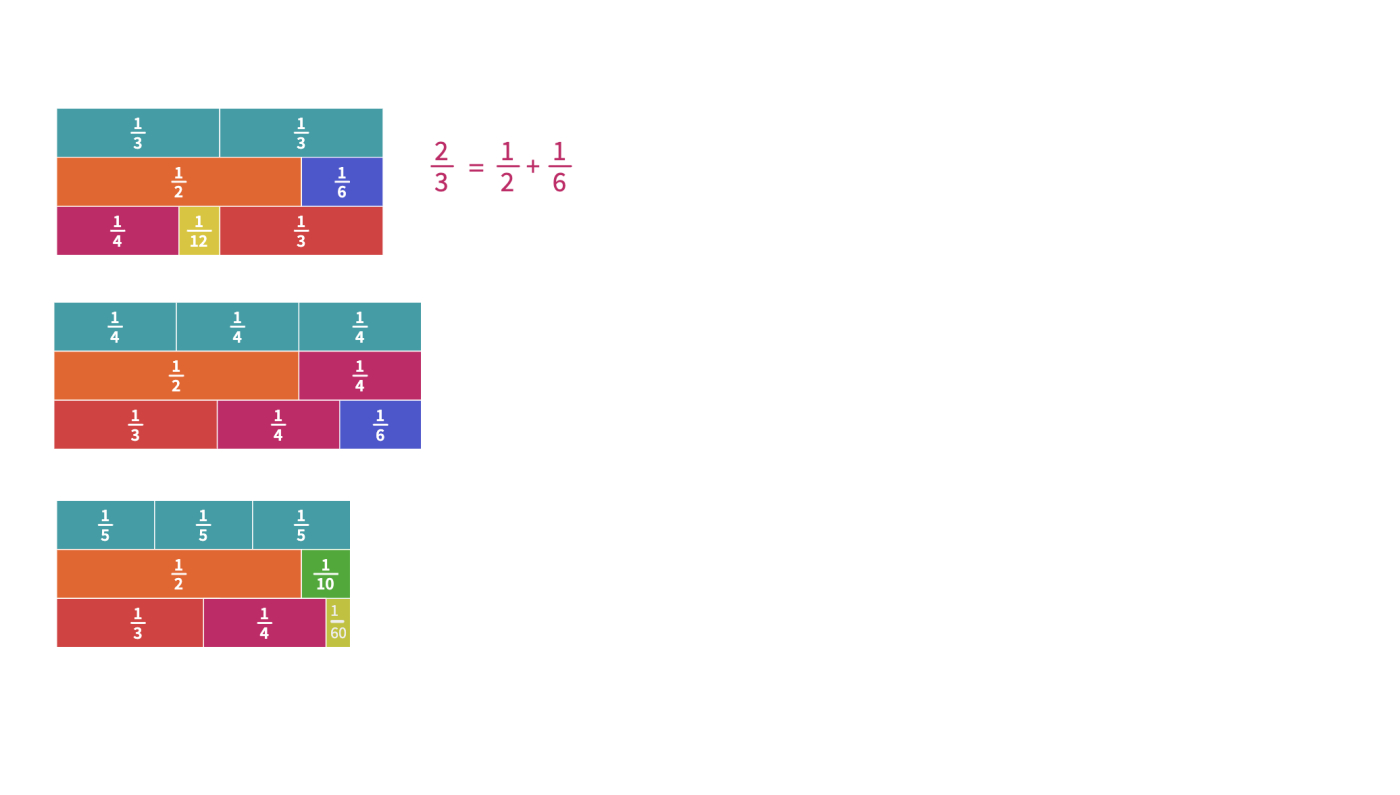
Students can use fraction bars to show the first few fractions. Remind them to use the "Rename" button on the center bottom toolbar to create more unit fractions up to $\frac{1}{24}$.

Encourage students to find as many different sums as possible as well as the one with the least possible number of unit fractions.

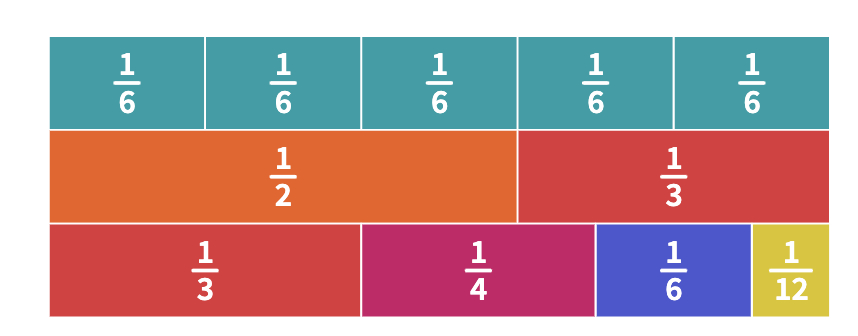
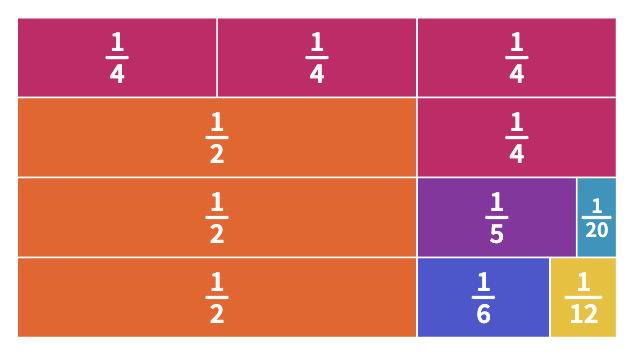
Also, suggest them to work systematically and express each fraction starting from $1/2$ as the sum of unit fractions. Here are some possible representations:

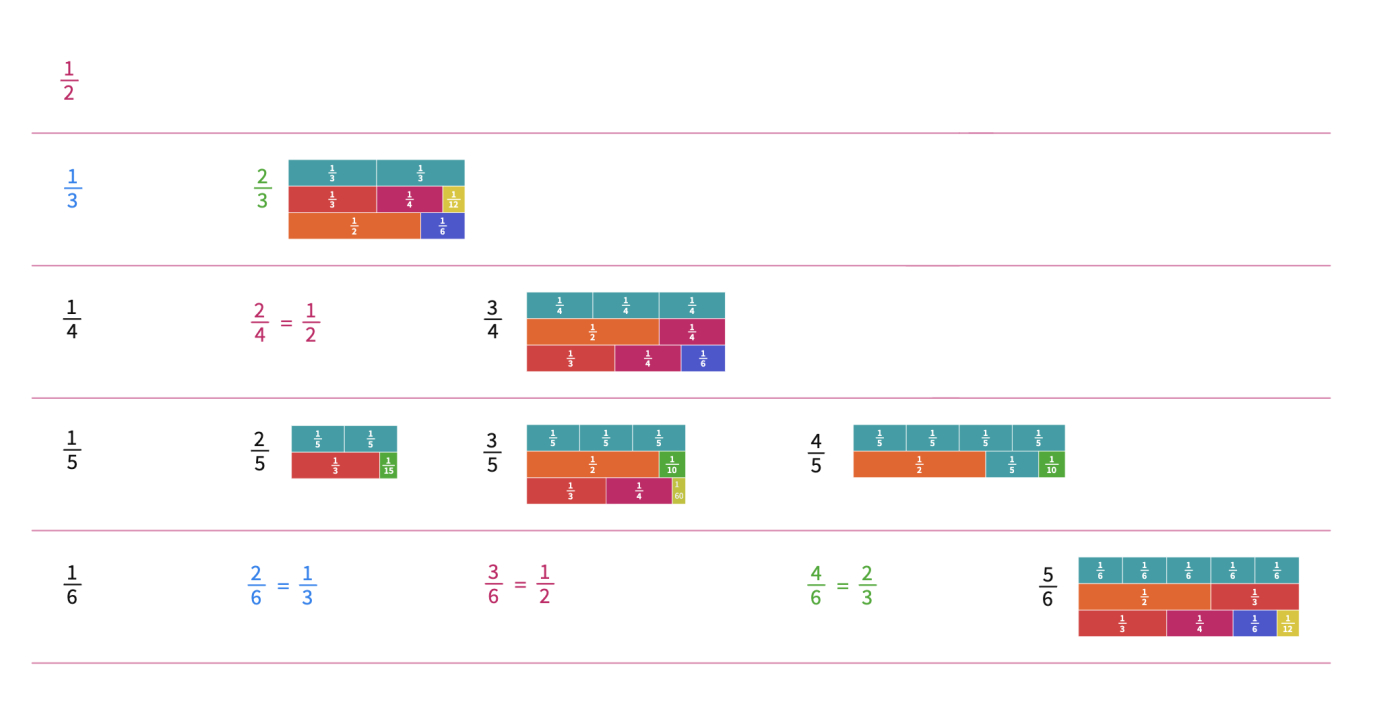
Take some time to discuss their findings, perhaps using the following prompts for discussion:

• How many different ways can they come up for writing the sums?
• Can every fraction be written by only using two unit fractions?
• Can they develop a strategy to find the unit fractions? What can be the best way of writing the fractions in the Egyptian Method?
  • To be able to express the original fraction with the least possible unit fractions, one needs to find the biggest unit fraction in it. Could they find a method for finding the biggest unit fraction?

Expressing fractions as the sum of the least number of unit fractions

Since the biggest unit fraction is $\frac{1}{2}$, some students might categorize fractions as bigger than or smaller than half. After subtracting the biggest possible unit fraction from the original fraction, they apply the same steps for the remaining part. In fact, this method is called Fibonacci's Greedy Algorithm. This is called the greedy algorithm since we (greedily) take the largest unit fraction we can and then repeat the same process on the remainder. The Greedy Algorithm sometimes fails to give the sum with the least number of unit fractions.

$\frac{7}{9}-\frac{1}{2}=\frac{5}{18}$

Here, the students might try different fraction bars to get closer to cover the remaining area.

$\frac{5}{18}-\frac{1}{4}=\frac{1}{36}$

So one way to express $\frac{7}{9}$ as the sum of unit fractions is $\frac{1}{2}+\frac{1}{4}+\frac{1}{36}$ and using this sum, Egyptians used the least number of symbols to carve $\frac{7}{9}$ in a stone.

Exploring different ways of writing the sum without any restrictions

Some students may realize for a unit fraction as $\frac{1}{n}$, $\frac{1}{n+1}$ is the biggest unit fraction that can be extracted from it to create other unit fractions using the remainder.

For example;

$\frac{1}{2}=\frac{1}{3}+\frac{1}{6}$

$\frac{1}{3}=\frac{1}{4}+\frac{1}{12}$

$\frac{1}{4}=\frac{1}{5}+\frac{1}{20}$

$\frac{1}{5}=\frac{1}{6}+\frac{1}{30}$

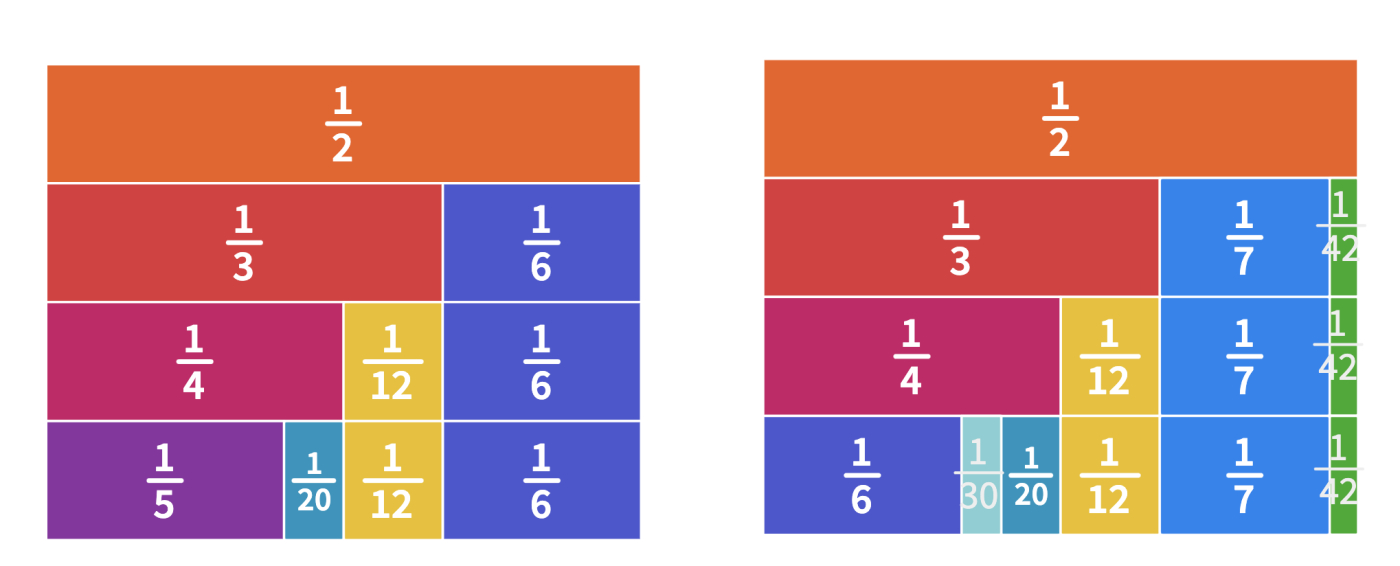
So any unit fraction can be replaced with infinitely many other options, which give infinitely many ways to write any fraction as the sum of unit fractions.

Closure

Invite students to share which approaches they found most useful when answering these questions. To close the lesson, as an exit card, you may ask the following questions.

• Is it possible to represent any unit fraction as a sum of two unit fractions? ( $\frac{1}{3}=\frac{1}{4}+\frac{1}{12}$)
• Can every fraction be represented as a sum of two unit fractions?
• The .......... of two unit fractions is always a unit fraction.

  a) sum b)difference c)product d)quotient

• We have already seen that $\frac{3}{4}=\frac{1}{2}+\frac{1}{4}$.
  • Can you write $\frac{3}{4}$ as $\frac{1}{2}+\frac{1}{5}+\frac{1}{?}$
  • What about $\frac{3}{4}$ as $\frac{1}{2}+\frac{1}{6}+\frac{1}{?}$.
  • How many more can you find?

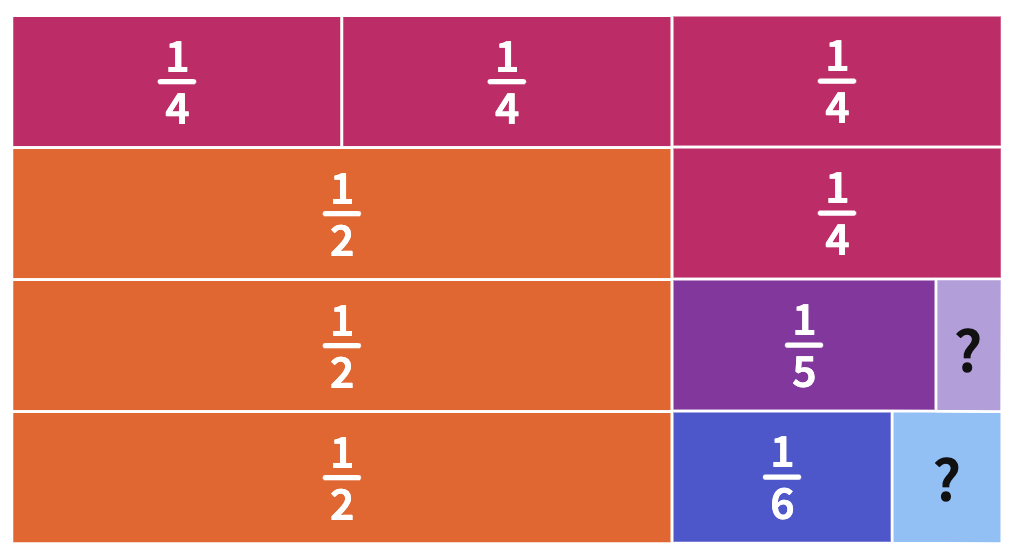
Answers:

• Yes. All unit fractions can be written as the sum of two unit fractions.
• No. Not all fractions can be written as the sum of two unit fractions. For example $\frac{4}{5}$ is written as $\frac{1}{2}+\frac{1}{5}+\frac{1}{10}$. Therefore, it can only be written as the sum of at least three different unit fractions.
• C. Only the product of two unit fractions always gives another unit fraction.

• $\frac{3}{4}$ = $\frac{1}{2}+\frac{1}{5}+\frac{1}{20}$ and

  $\frac{3}{4}$ = $\frac{1}{2}+\frac{1}{6}+\frac{1}{12}$ . We can write $\frac{3}{4}$ as the sum of unit fractions in infinitely many ways. Each fraction has an infinite number of Egyptian fraction forms

Support and Extension

For students ready for additional extension in this lesson, consider asking questions;

• $\frac{1}{2}=\frac{1}{3}+\frac{1}{6}$, $\frac{1}{3}=\frac{1}{4}+\frac{1}{12}$ ... by following this pattern, how can we write a general rule for every unit fraction a representation as a sum of two different unit fractions.
  • $\frac{1}{n}= \frac{1}{n+1}+\frac{1}{n(n+1)}$

Older students may also work on similar generalizations for finding all the possible unit fraction pairs.

$\frac{1}{n}= \frac{1}{n+a} + \frac{1}{n+b}$ where $a.b=n^2$ For example for $\frac{1}{6}$ where $n^2$ is 36, $(a,b$) can be ($1,36), (2,18), (3,12),(4,9),(6,6)$

$\frac 1 6$ = $\frac{1}{7}+\frac{1}{42}$

$\frac{1}{6}=\frac{1}{8}+\frac{1}{24}$

$\frac{1}{6}=\frac{1}{9}+\frac{1}{18}$

$\frac{1}{6}=\frac{1}{10}+\frac{1}{15}$

$\frac{1}{6}=\frac{1}{12}+\frac{1}{12}$

Older students can also explore why Fibonacci's Greedy Algorithm does not work for finding Egyptian Fractions using the examples like $5/21$.

For students needing additional support with these ideas, rather than focusing on the general rules, the number of numerical examples can be increased so that they can find the largest unit fraction that can be extracted from the original fraction and work on the remainder by using the same method. In this way, without writing the general rules algebraically, they can still prove that any fraction can be written as the sum of unit fractions in infinitely many ways.

Polypads for This Lesson

To assign these to your classes in Mathigon, save a copy to your Mathigon account. Click here to learn how to share Polypads with students and how to view their work.

Desmos Classroom Link