Part 1: Regular Dodecagons
Regular Dodecagons are 12-sided polygons with interior angles of 150° and exterior angles of 30°. In this activity, we are going to create regular dodecagons by using rhombuses.
Try it out! Can you use the 3 different rhombuses on the canvas below to fill up the dodecagon? Copy the shapes as needed.
For the dodecagons you have created:
- How many total rhombuses did you use? (Recall that all shapes above are rhombuses)
- How many of them were also squares? parallelograms?
- What are the angles of the different rhombuses you used?
- Measure the interior angles of rhombuses. Think about the different ways that they can come together to create each interior angle of the dodecagon
Part 2: The smallest number of rhombuses
What is the smallest number of rhombuses you can use to create a dodecagon? The dodecagon will be smaller than the one you made above
Compare the dodecagon designs that you can create with the minimum number of rhombuses:
- How many tiles did you use in each?
- How many possible arrangements are there?
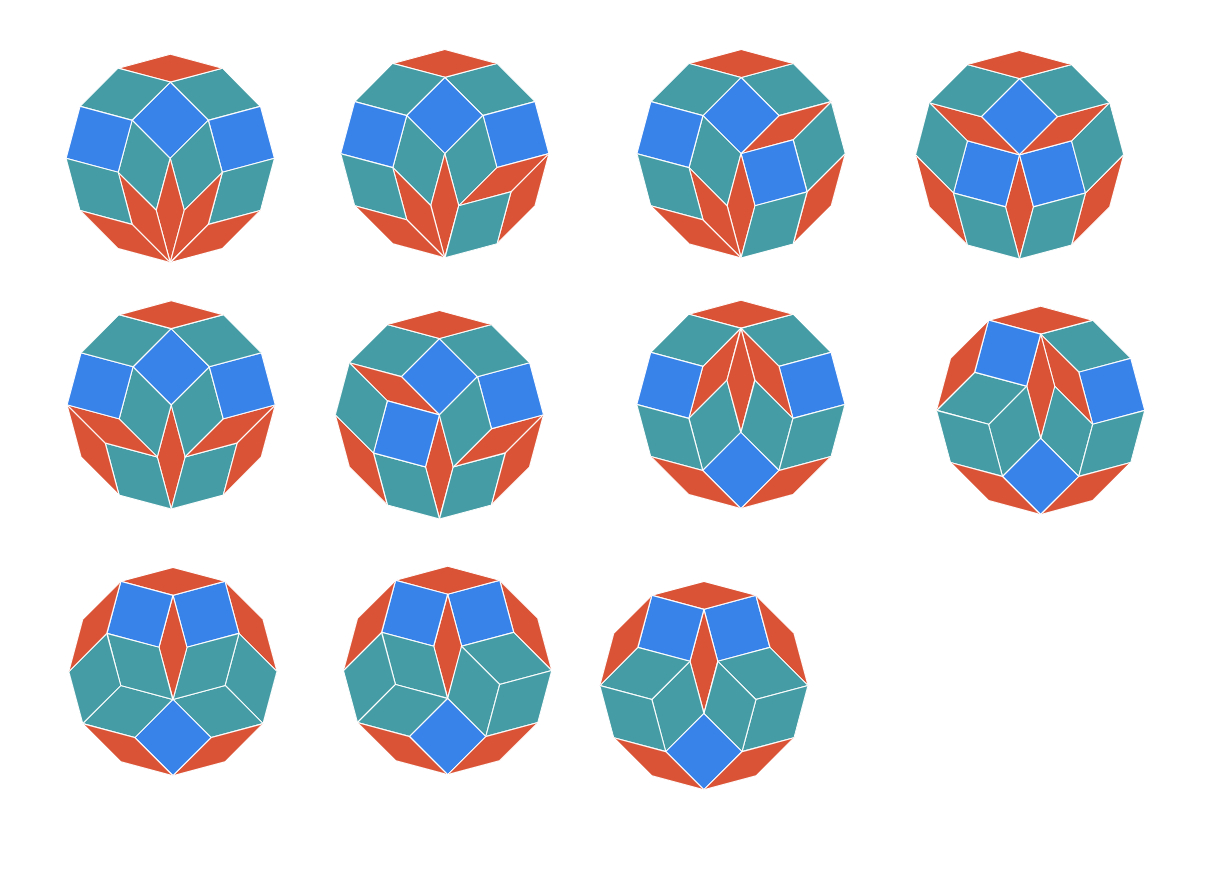
Solution
When using 15 quadrilaterals, there are 11 ways to create a regular dodecagon:
Extensions
- Explore the different decompositions of 4 -24 sided polygons
- Lindgren's Symmetrical Decompositions of Regular 2n-Gons