Lesson Outline and Objective
Students will understand how to move between the forms and for a quadratic expression.
Algebra tiles are a powerful tool for helping students make the connection between symbolic manipulation and geometrical representation using area models. In this activity, students can explore different algebraic manipulation techniques using the Polypad algebra tiles to represent expanding brackets and factorising. This task may take more than one lesson, depending on students’ prior experience with algebraic manipulation; it is worth taking the time to really embed the area model for understanding the different representations. Using algebra tiles and the area model provides a concrete connection for students who may find manipulation of abstract expressions challenging.
Warm Up
Optional Review of Area Model of Multiplication
If your students could benefit from a review of the area model of multiplication, consider solving 27 x 15 in three different ways using this Polypad canvas:
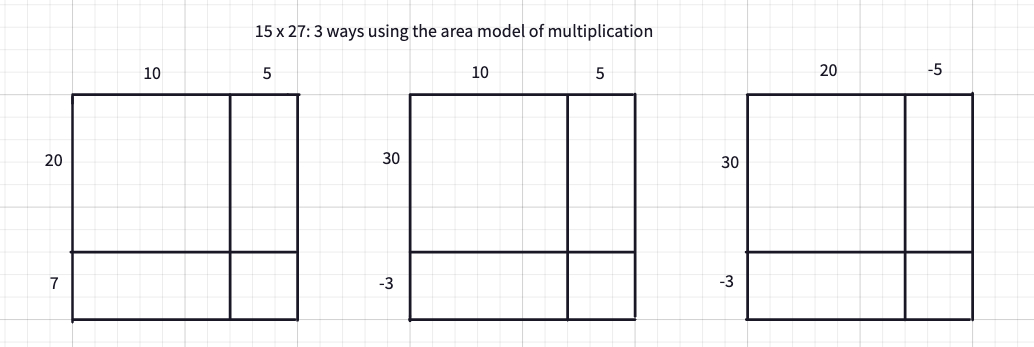
This can also serve as a good review of multiplication of negative numbers. This Polypad canvas shows the solutions:

Expanding a Single Bracket
Share this Polypad canvas with students. This warm-up introduces the use of algebra tiles for multiplication in the area model. Consider working on it as a class together or having students work on it small groups on their own devices

The correct labels are as follows:

Main Activity
Start the main part of the lesson by showing how the algebra tiles can be used to expand double brackets:

Ask students to explain how this diagram shows that . Then invite them to create representations for some other sets of double brackets. Students can either make up their own examples to explore or you can provide them with examples. Remind students to take care when working with negatives as well as positives. The algebra tiles include a -1 tile and a -x tile; the x and -x tiles can be moved over each other and greyed out as shown. This helps students with collecting like terms when there are both positives and negatives.
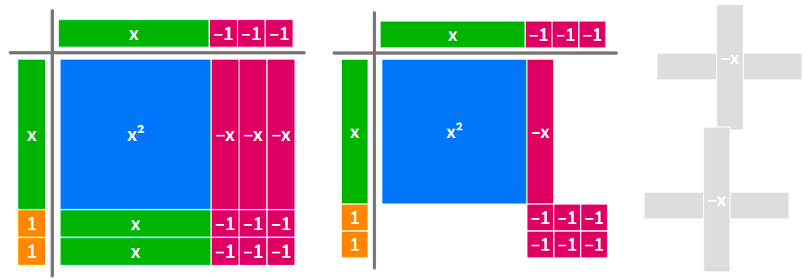
As part of their explorations, it’s worth encouraging students to explore families such as and .
Next, introduce using the algebra tiles to explore factorization. One way to do this is to give them the results of expanding double brackets and challenge them to work out what you might have started with.
This canvas has arranged in a rectangle so that students can spot the two factors.
This canvas presents , but not arranged, so students will need to rearrange the pieces into a rectangle and then find the factors. Students can use number tiles to help them find arrays of whole numbers if needed. See this video for an example of the factorization of .
Students can create questions for each other by expanding a pair of brackets and then challenging their partner to work out what brackets they expanded. There are opportunities for fruitful discussion about what each part of the expanded form of the quadratic tells us about the factorised form, and how we can work systematically through combinations which add to give the coefficient of x until we find a pair whose product gives us the constant term.
It is worth drawing attention to the signs of the different terms: if , students could be encouraged to explore when b and c are negative by trying combinations of positive and negative p and q, and then using the algebra tiles to explain their conjectures.
Support and Extension
The use of algebra tiles makes a real difference as a concrete representation for students who find it difficult to manipulate quadratics. Start with examples that just use positives before introducing examples with positive and negative numbers, and allow plenty of time for creating diagrams before encouraging students to move to diagrams and then perhaps a grid method on paper. For example, they might express like this:

Each section of the grid now has an algebraic expression instead of the algebra tiles.
One possible extension activity is to invite students to explore expanding, and then factorizing, non-monic quadratics. Start by sharing this Polypad and having students expand the expression. Then, ask students to use Polypad to factor non-monic expressions. One example could be to factor .
For another extension, ask students to use Polypad to factor the expression . Students will discover that this expression is not able to factored. Conversation can ensue around which quadratic expressions can be factored and which cannot. Students could use a random number generator to create whole number values between 1 and 50 (or any number range) for b and c in . Students can record what percentage of them are able to be factored. Consider also using the activity at this Polypad. Depending on where you are in the algebra curriculum, you could conclude this discussion by sharing with students that you’ll be learning about other ways to handle quadratic functions that are unable to be factored.