Overview and Objective
The Spiral of Theodorus is a shape composed of right triangles, placed edge-to-edge. In this lesson, students explore the spiral. While drawing the spiral, students will repeatedly construct perpendicular lines. For some students, this repetitive process can lead to creating their specialized geometrical tool.’’
After the construction, students will apply the Pythagorean Theorem to calculate the hypotenuse of each triangle. After finding the lengths, they use the ruler to measure these lengths to estimate the value of each square root. Then, they approximate their location on a number line diagram.
Warm-Up
Start by asking students to construct a right isosceles triangle with right sides of 1 cm using the segment tool at the bottom of the Polypad canvas. They may select the square grid to construct their triangle or use the ruler to construct 1 cm-long right sides. There are many more ways to construct the first triangle. Ask students the length of the hypotenuse of the triangle.
The Pythagorean Theorem tells us the length of the hypotenuse is cm. Invite one of them to use the ruler to measure the length of the hypotenuse. The decimal value of will be around
You may also use the number line, to locate √2. Here, you may discuss the difference between rational and irrational numbers perhaps by using the story of Pythagoras and Hippasus.
√2 is an irrational number like π. It has a non-terminating and non-repeating decimal expansion.
√2 1.414213562373...

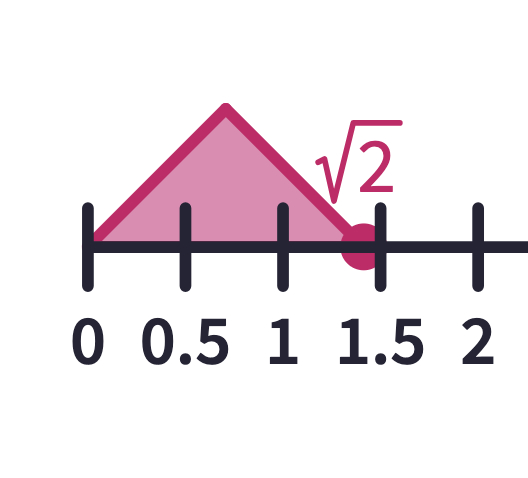
Although we do not know the exact decimal expansion, we can still locate on the number line since it can be constructed geometrically (using a compass and straightedge). Such numbers are called constructible numbers. Ask students if they think they can construct other square roots like √3, √5, √6, √7 to approximate their decimal expansion.
Give students some time to think about the construction of √3 first. After sharing possible methods to create √3, you may start introducing the idea of the Spiral of Theodorus.
Main Activity
Theodorus of Cyrene was an ancient Greek who lived during the 5th century BCE. The spiral of Theodorus is a shape composed of right triangles. It starts with the isosceles right triangle and adds another right triangle with the right sides of 1 cm and the hypotenuse of the previous one.
In this way, the second triangle will have a hypotenuse of
cm
When we add another triangle;
cm and another,
cm ...
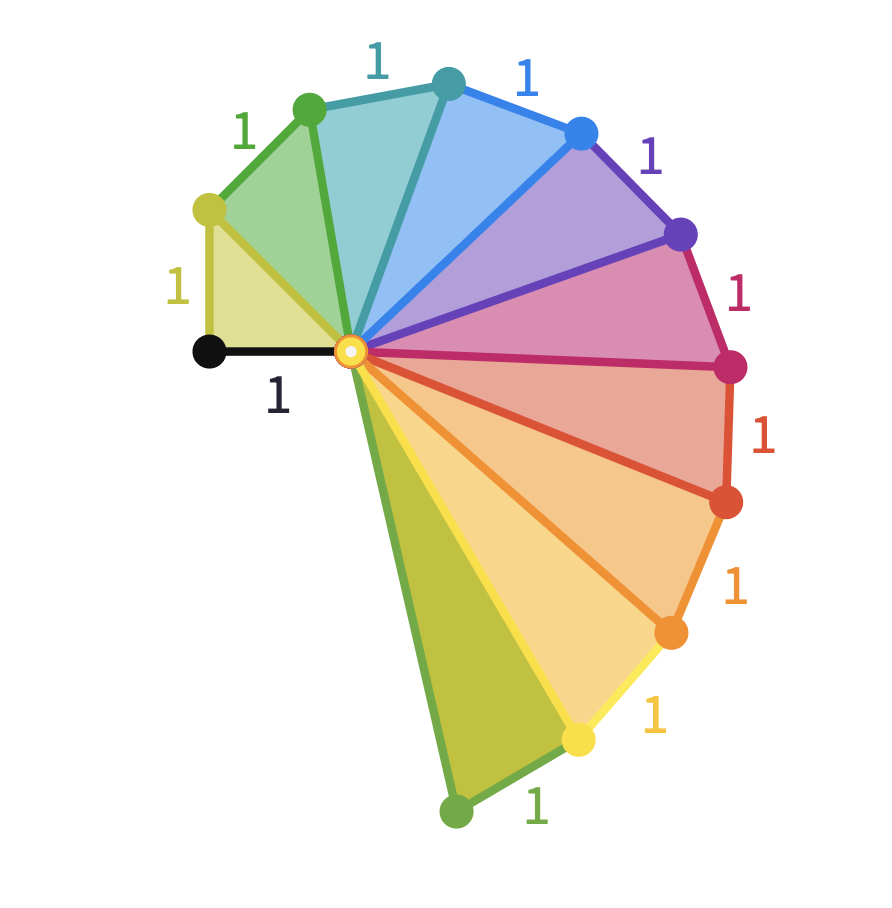
So algebraically we now know that we can construct the square roots of all positive integers using the right triangle method.

To be able to make accurate measurements, we need to construct the triangles carefully.
The goal is to add another right triangle whose one right leg is the hypotenuse of the first triangle ( cm), and the other right leg is 1 cm. Ask students the possible strategies to make this second step of the drawing.
Since using square grids will not work anymore, they may use the protractor and ruler to construct the new right triangle. This method may take time but works perfectly for the construction of the spiral.
Some students might use the bottom center toolbar to create perpendicular lines. In the extension part, creating a specialized geometric tool for the iterative process of adding a right triangle is shown as another method. Click here to learn more about the geometric construction tools in Polypad.
The original spiral stopped with a triangle with a hypotenuse of . But you may extend the triangles as much as you want. After completing the spiral, let students calculate the length of each hypotenuse using the pattern of the Pythagorean Theorem.
Clarify with the students that given a compass and a straight edge, one can construct the square root of any counting number. Invite students to share their different designs of the spiral and any mathematical questions that might have occurred to them.
Closure
To close the lesson, share this canvas with the students and let students measure each hypotenuse with a ruler to come up with the approximation of each square root.
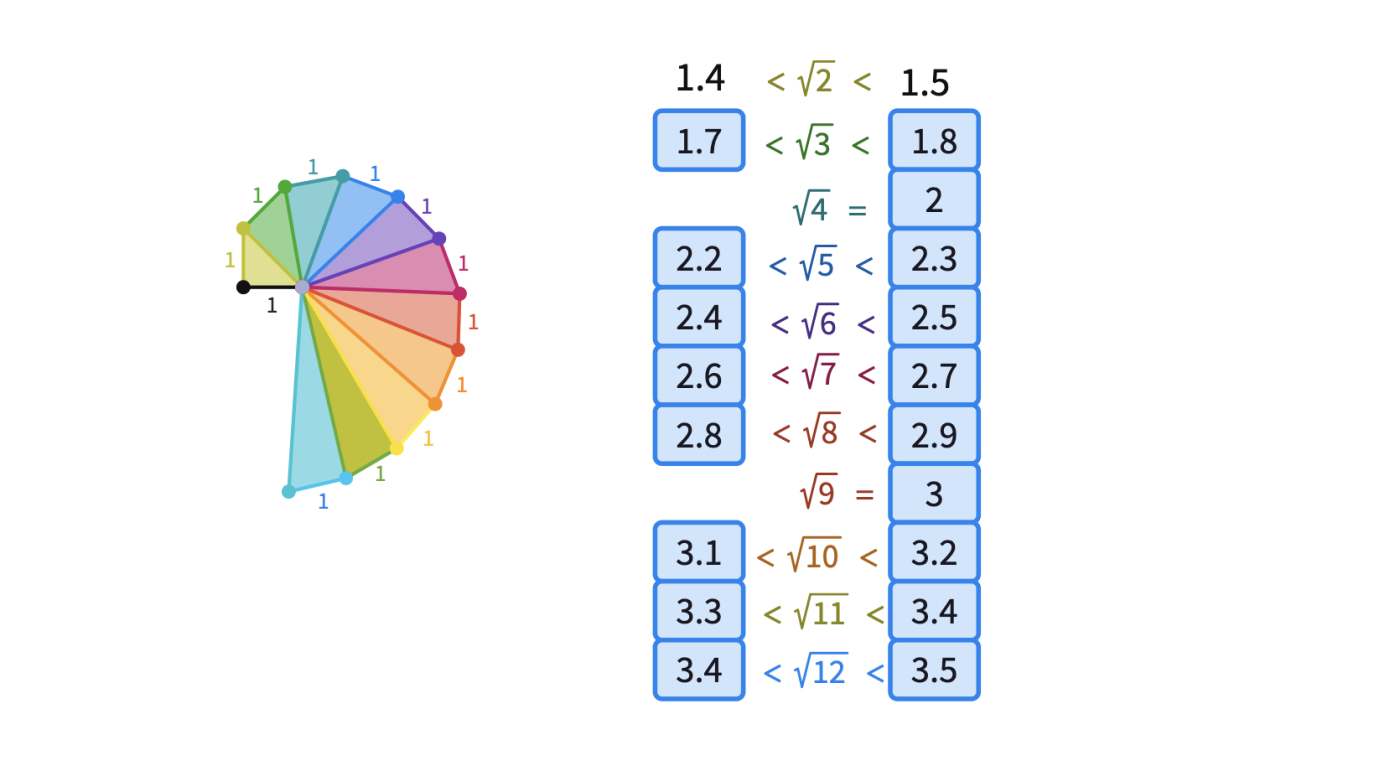
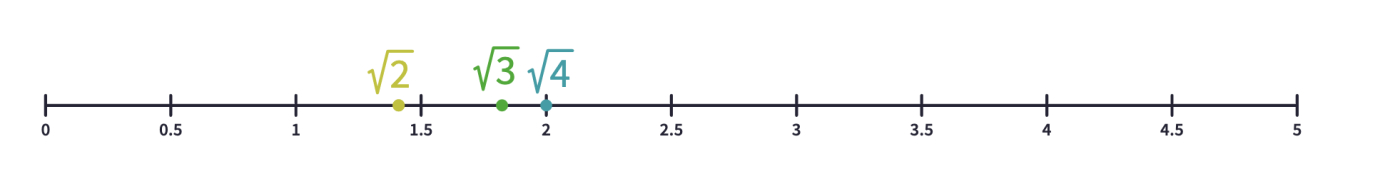
As a whole class extension, after finding the approximations, students locate the square roots on the number line. This may lead to the discussion of techniques for estimating the values of the square roots without actually constructing and measuring them.
They may realize the perfect squares that are above and below the given square root.
For instance,
< <
and since 11 is closer to 9 then it is to 16, we can estimate the decimal value of as less than .
Support and Extension
For students ready for additional extension in this lesson, consider asking to create a specialized tool for the iterative process of creating Theodorus Spiral.
For the older students who have a background with trigonometry and limits, you may also ask about the growth rate of the spiral.
The spiral can also be used to provide a creative solution to the problem of how to divide a circle into 5 equal areas.
Begin by creating the radius of the first circle, which is . From there, just by creating the rest of the spiral, we can create the other radii of the larger circles. What is the area of the pink circle? What is the area of each region between the co-centric circles?

For students needing additional support with these ideas, the Spiral of Theodorus canvas can be shared directly without them working on the construction process but rather concentrating on measuring the lengths and estimating the decimal approximations of the square roots.
Polypads for This Lesson
To assign these to your classes in Mathigon, save a copy to your Mathigon account. Click here to learn how to share Polypads with students and how to view their work.