Lesson Outline and Objective
Students will compare fractions with different denominators.
Fraction bars offer a great concrete tool for working with equivalent fractions. This task encourages students to move from the concrete to the abstract by creating equations and inequalities with fraction bars and then explaining numerically what is happening. Polypad provides a safe place to explore and for students to generate their own examples at a level they are comfortable with, before gradually pushing themselves to create more complicated examples as they become more confident.
Warm Up
Show students this fraction wall built in Polypad:
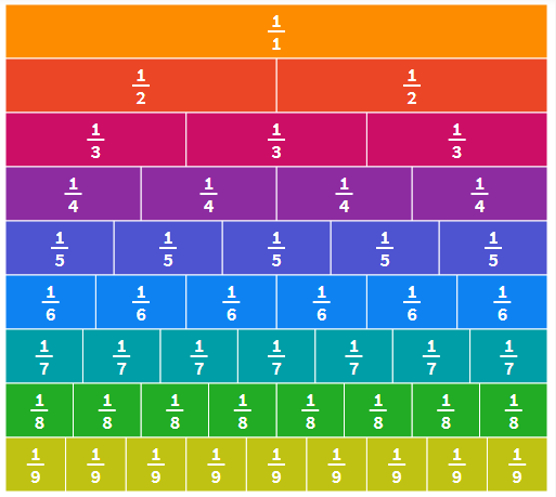
Invite them to write down as many sets of equivalent fractions as they can see in the picture. For example. they could write because the line for four of the groups of coincides with the line for two of the groups of .
Students who complete this quickly could also write down other equivalent fraction relationships that do not appear on the wall by using denominators bigger than 10, or fractions bigger than 1. Encourage struggling students to use the bars to create equivalent fractions.
Once students have created their sets of equivalent fractions, take some time to talk about how they can check their answers numerically. If your students would benefit from a more concrete review of this idea, you could create something like this Polypad with them.
Main Activity
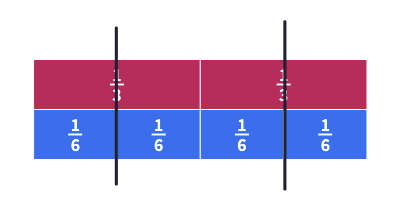
Show students the picture below and pose the question: Which is bigger: or ? By how much?
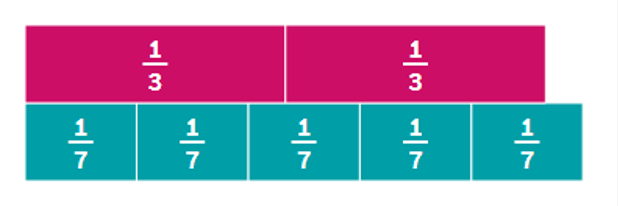
Students are likely to agree from the picture that it is clear that , but it’s worth pointing out that it might not always be so obvious, and that students won’t always be able to use Polypad to help them! Invite students to suggest ways of working out which is bigger, and by how much. Use the pen tool to demonstrate dividing each third into seven pieces, and each seventh into three pieces:
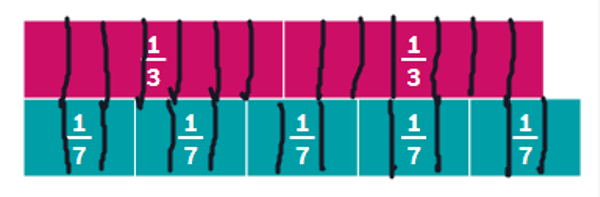
Ask students if they can now make a better estimate of how much bigger is than . It may be somewhat clear that the bars differ by 1/21. Confirm this by showing the following: and , so not only can we work out that , we can also work out that . As you work this out, you could label the Polypad as shown:
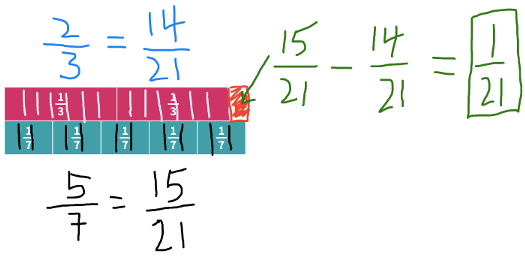
Share with students that the visual of and made it clear which fraction was bigger and that drawing in the lines to represent equivalent fractions also helped show the difference of .
Next, invite students to create some fraction inequalities of their own. Consider any of the following options:
- Some students might just want to explore fraction combinations without a specific goal or challenge in mind. Invite them to label their diagram as shown above and share their Polypad link with you when finished. Maybe they’ll be ready now to move onto some of the challenges below.
- Challenge students to create examples in which the fractions differ by a certain amount. Make a list of unit fractions on the board ( and so on) and challenge the class to find as many as they can. Check them off as students find solutions. Students can show their work on Polypad as shown above and share their link with you.
- Challenge students to create examples of equivalent fractions in which each row uses more than 1 type of unit fractions (The top and bottom fraction bar must have at least two different colors).
- Challenge students to create examples in which the two fraction representations are as close to each as possible, but not equivalent. Share with the class that based on the example above with 3rd and 7ths, the smallest difference the class has found so far is . Challenge them to beat this.
Below are some examples of smaller ones. The 1st one has a difference of . The 2nd one has a difference of . The 3rd one has a difference of . Use these to provide hints to students as needed.
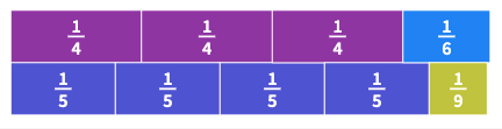

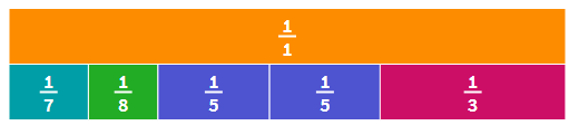
Support and Extension
To get students used to the idea of making inequalities with fractions, invite them to build a fraction wall of their own, and write down all the different inequalities they can make with it. Encourage reasoning that will help them to develop number sense and estimation skills – for example, when comparing with , invite students to consider how each fraction would compare with . Students might also benefit from thinking about what happens when we compare fractions with the same numerator, but different denominator.